Toothpicks Activity
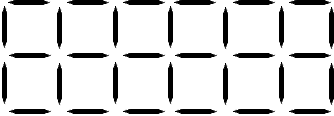
Directions:
- Use toothpicks to make the pattern.
- Select a strategy to find how many tooth picks are in the pattern without counting each toothpick?
- Write an equation to illustrate a strategy to determine the number of tooth picks. You may change your strategy.
- Exchange an equation with a partner. Prove to yourself the strategy you were given will determine the number of toothpicks.
- Return the equation to the partner and explain strategies to each other.
- Form groups of four and repeat steps 5 and 6.
- Everyone puts their equation on the board.
- Each person tries to understand how each equation on the board is a strategy to find a solution.
- Take turns listening and explaining the equations on the board until everyone understands the strategy for each.
- Organize the equations into categories.
- Each person, pair, or group of four creates a new problem using what was learned today.
- Each person or group shares a new problem with the others.
- Which equation was the most unique? Why?
- Each person or group shares what equation they think is most unique and why it's most unique with the others.
Hint: Look for patterns.
For example 6 C's (each C made with 3 toothpicks) and one toothpick at the end of the row make the top row of squares. Bottom row 6 L's plus one.
Discussion: Organize all the equations in some kind of order.
Mind boggler:
Is this a function or an equation or both?
Equation. x + 3 = 10; only one solution. An equation is only one way to express a relationship.
Function. For F(x) there is only one solution (unique answer). A function is a relationship between two variables such that every value of the independent variable, within the domain of the function, maps to one value for the dependent variable.
Functions can pass a verticle line test (a visual way to determine if a curve is a graph of a function or not by seeing if the line passes through multiple y points above the same point on x. If it does, then there are more than one solution. For example, a graph in the shape of the letter S would have three points above one or more of the same x point.
Both Equation and Function. x = 2y + 3
Not all functions can be expressed in any familiar way as an equation, but all functions can be used to make equations.
Enjoy!